Single Slit Diffraction for A Level Physics

-

 

Single slit diffraction for A Level physics
Credit: Olympus



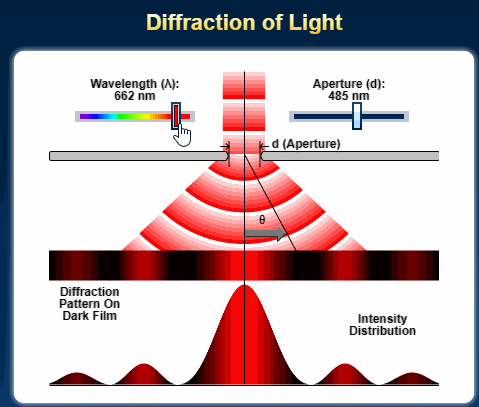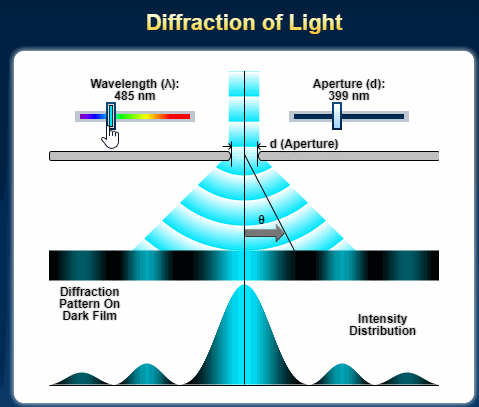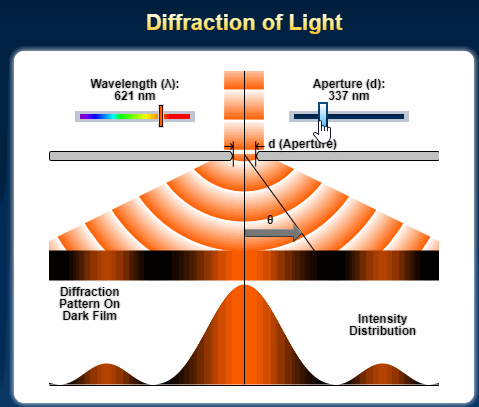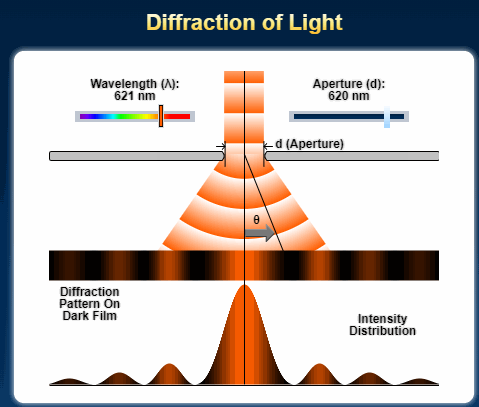
The diffraction of a beam of light through a single slit is given by the following equation.

x = d sin θ, where x is the path difference and θ is the deviation of the path as an angle. d is the width of the slit or aperture. 

Single slit diffraction - maths
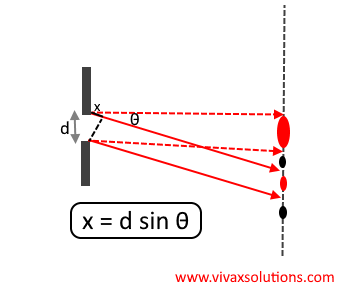Single slit diffraction


The greater the angle of diffraction, the greater the value of x.

If x is a multiple of λ, the wavelength, bright spots are visible on the screen. If x is a multiple of 1/2 λ, however, the spots become dark; at those points, constructive and destructive interference take place respectively. 

 

 


The spot spread on the screen with an equal gap between them.

The values of x for the bright spots can be λ, 2λ, 3λ and so on. For the dark spots, the are 1/2 λ, 3/2 λ, 5/2 λ and so on. 

The intensity of the spots, however, goes down with the distance from the central spot, which is twice as wide as other spots.

From the above equation, 
sin θ = λ/d

Based on the above formula, the greater the width of aperture, the smaller the angle of diffraction. On the other hand, the smaller the width of the aperture, the greater the diffraction.

For the first bright spot on the screen, x = λ
sin t = λ / d
When diffraction is maximum in theory, sin t = 1
1 = λ / d
d = λ
When diffraction is maximum, the width of the aperture must be equal to the wavelength of the light.







Comments

Post a Comment

Popular posts from this blog

Phase Difference between Two Points on a Wave and Path Difference Explained - interactive

-
Image
  In the above animation, five points on the wave are considered for the explanation.  The fully interactive applet is given below for you to practise. The phase of a point implies its direction of vibration on a wave. For example, both points A and E vibrate exactly the same way are said to be in phase: when A goes up so does B; the phase difference is either 0 0 or 360 0 . If you consider the motion of points A and C, on the other hand, when A goes down C goes up or vice versa. Therefore, A and C are said to be out of phase; the phase difference is 180 0 . That means the phase difference between two points on a wave - or two waves for that matter - can take any value between 0 0 and 360 0 .  The phase difference between points A and B, for instance, is 90 0 . From the above examples, it is clear there is a connection between the path difference between two points - or two waves - and phase difference. It is as follows: Phase difference = (path difference / wavelength) x 360 φ = x
Read more

Frequency Amplitude and Wavelength of a Transverse Wave - simulation

-
Image
Waves A wave carries energy from a point to another point in a medium / vacuum without the movement of the particles along with it. In the above animation, the wave moves from the left to the right; the particles, however, just vibrate vertically without moving along with the wave. Transverse Waves If the direction of the wave is perpendicular to the vibration of particles, it is called a transverse wave. E.g. Water waves, radio waves, microwaves Amplitude The maximum displacement of a particle in a wave is called amplitude. Intensity / loudness The intensity / loudness of a wave is proportional to the square of the amplitude. The greater the amplitude, the greater the intensity / loudness. Amplifier This is what an amplifier does: it increases the amplitude of an input signal, which in turn leads to the increased loudness or intensity. Frequency  - f The number of cycles of a wave that passes through a point in a unit time is called frequency. Wavelength  - λ The distance between
Read more